Grading in Thinking Classroom – #Blaugust Post 1

Here we go! Haven’t been on this page in a long time! Not sure I have something interesting to say today or all the days of August…but we will see.

Last summer I read Building Thinking Classrooms by Peter Liljedahl, and it changed how I approached everything in my class! I knew I had to reread it this summer because there was something missing in my class. I was pretty faithful to following the first 3 toolkits that are discussed in the book, but not the 4th on grading. In rereading the book I found a few things I could improve upon this year with what I did last year…but the grading aspect I think will revolutionize my thinking classroom. I cannot recommend this book enough! The issue I had with my classes was a majority of my student’s time in class was spent at the whiteboards working with random groups, but nothing in their grade reflects that. Grade what you value is a macro move in Chapter 12, so that’s what I’m going to do. I created a sheet to share with students that will illustrate how they know if they know a goal and how well.

I then made one for myself to be sure I don’t miss an opportunity to give students credit for work they do.

I won’t get in the weeds about how to get a grade out of this, that’s what the book is for. I’ll just say that this is what was missing from my class last year…especially in AP Calculus. It’s important that the students understand the material, not just get As on quizzes. This is a step closer to this goal and value for me.

#myfavfriday

What a long week…every Friday I feel it. There were some great moments this week though.

On Tuesday, the 9th graders in my Honors Geometry were at an assembly. Just enough kids that would make it a pain to move on with the remaining 8th graders (they come up from the 3 middle schools to take the course a year early). I was at first struggling with the thought of what to do…and then MTBOS! Of course we would spend some time with WODB and counting dots! This group of kids got into it! They were all geeking out over it, not worrying about others thinking they weren’t cool. Love that these kids have a place to enjoy math!

In AP, we were working with the definition of the derivative. Since it’s BC, chances of them seeing this on their test are low. They do need to recognize the functions and the difference between average and instantaneous rate of change. On Facebook someone posted a picture of an activity that looked intriguing to me as an opener. Rather than rewriting the cards every year, I typed the expressions on “cards” and I plan to laminate them when I get a chance. It turned out to be an ok activity 2nd hour…but so much better 3rd as usual after an hour of experience!!

Cards

Yesterday, as part of my basic derivatives lesson we worked on this Desmos activity to “discover” the derivative of sine and cosine. The kids found it very useful to remember how those derivatives happen!

Sine Cosine

I forget where I found the links but I know it was in the #mtbos.

And to top the week off…Math with Bad Drawings came in the mail today! I had a stack of papers to check, but not sure I’m going to get to them now!!

#MTBoSBlogsplosion Round #3

Better late than never, right?! I read the prompt for this week’s Exploring the MathTwitterBlogosphere and thought…who is spying on me? Has the MTBoS found a way to get in my mind?! Next week, I will start teaching Honors Trigonometry for the first time in 2 years. I was looking over my old Interactive Notebook and realized I had only used the INB idea once for honors trigonometry, so some of the pages could use a makeover. Off to Pinterest I go! My colleague and I share a Trigonometry page so I wanted to see what was there.
A few minutes on Pinterest led me to Sarah’s Math = Love site and Shireen’s Math Teacher Mambo blog! Great blogs, if you have never been! They both have fabulous ideas for my beginning Trigonometry unit. The pages I used the first time teaching this course with an INB are not bad, but I like to spice things up with some fresh ideas.

The first unit in trig consists of a lot of angle basics necessary for the rest of trig. First, I saved an idea from the agony and dx/dt‘s page to explore what a radian is. I have done this activity before, or one similar…with string. How boring is string when you can use candy!!!!

Twizzler’s Pull and Peel to be exact. I love using food whenever I can, and don’t know why I didn’t think of that! I will probably do something like Sarah’s pipe cleaner idea when transferring the lesson to their notebooks, candy doesn’t last on paper.

Instead of my boring sketching angles pages, I am going to use the cool page Sarah created. I even get to use a fastener so the angle rotates in the notebook! Oh boy oh boy oh boy!

What drew me to Math=Love’s trig page was her Coterminal Angles Sort. So that will be next in my notebook.

The coolest thing ever in getting students to understand reference triangles and special angles though might be from Math Teacher Mambo, and I am so excited to give it a try and see if my students think as highly of it as I do! I love how colorful this all is…and I especially like doing something that helps with student’s understanding of fractions.

That is as far as my thoughts have gone considering I am not yet done with this semester and have some planning yet to do with my current classes. The MTBoS is so helpful and I love that these teachers have provided links to the handouts. Things I create will too be uploaded here…as the unit goes. It is so tough to choose just one thing to write about as far as the usefulness of this community, I utilize so many great ideas every day and try to make them my own. Please know I appreciate all of your blogs so much for inspiring me. Thank you for sharing your ideas!!

Ice Day, Christmas Break, 2 Snow Days and Maybe More?!

It has been a while! The title of this post pretty much sums up what I’ve been doing, a whole lot of nothing (in regards to school!). I also noticed that there are a few things I missed in my posts from last year, you know, a couple of weeks ago!

Here are some things from my INB in Honors Precalculus…

We worked on the binomial theorem, and Pascal’s triangle before break. Then I had 2 days to talk about rational functions, which leads us into limits with Honors Precalculus (Pre-AP) so I searched the blogs and this is why the MTBoS is so fantastic…of course Rebecka had exactly what I was looking for! This note sheet worked perfectly to review rational functions with my students.

We were then supposed to have a test the Friday before break, but my title tells what is going on with that! I think my notebook is going to come in handy when we get back to school (whenever that may be). Students will be able to look through that to remind them of what we were learning before the extended vacation. A lot of modifications are going to have to be made to the rest of my curriculum though…exams are supposed to be the end of next week! What do you do when your semester is suddenly cut short? We are on block scheduling, and are done with these classes at the end of next week…

MTBoS Mission #4 – Listen and Learn (and Day 45)

Yesterday, I finally got around to some professional development at Global Math Department. I have watched a Global Math Department conference on Interactive Notebooks over the summer. It was actually my introduction to the MTBoS! From the Global Math Conference, I found links to people’s blogs, links to twitter, a whole new world of online math improvement! I loved the Interactive Notebooks so much, all of my classes are now using them! Through this week’s mission, I found that there was a conference on Foldables! I have developed a love for foldables this year, in case you couldn’t tell by past posts, so I was thrilled to get some new ideas from @jreulbach.

From the conference, I clicked on Julie’s wiki link where I found some templates for new foldables. What a find, because I am starting a new term, and students are always excited to see a new way to present the material. The templates I found used PowerPoint to create the foldables. Why hadn’t I thought of that before?! It is so much easier to get everything to line up the way I want using PowerPoint versus Word! This led to putting together my all new Chain Rule lesson I used today in Calculus. Well, this and a fellow bloggers – Rebecka and Sam.

Sam, through his post, helped me to develop the idea of outer and inner functions (so much easier than all of the letters students usually get lost in!), which helped me to create this introduction paper:

               

I then handed out notes to the students, the notes I created after watching the Global Math Conference on foldables.

     

We worked on the top flaps (left picture) together, as well as discussed the outer functions and their derivatives. This was all leading to an activity I found via Rebecka. I handed out cards to students in groups of 2 and after a short while, I had the students find the group with the same color writing to compare answers and to help complete the task, in case any of the groups were stuck.

Students then wrote their group’s information on a table projected to the entire class. From that table, and a few leading questions, students actually formed the chain rule! Actually, in my 3rd hour, I had a student state it pretty close to perfectly: “The derivative of the outer with the inner plugged in times the derivative of the outer”!! YES!! Awesome!!!

Then we discussed it a little more, having students try to explain in to their partner. I wrote on the board: y=f ‘ ( g(x) ) * g ‘ (x) and we discussed this, whether this made sense, and how this was similar to what the student said. Class time was just about up at this point, and I assigned 5 short problems for students to try using what we had discussed today. This will lead into tomorrow, where we will finish my foldable notes.

I was formulating the lesson since last week, prior to watching the Global Math Department post, but without viewing Julie’s conference, the lesson would not be put together in the same way. I can’t wait to have more time to watch more conferences…I really want to watch the Desmos one. Time is never on my side! Thank you to this fabulous community!

Personal Responsibility

How do you teach personal responsibility? Is it up to me to explain something until everyone “gets it”? Should I pause class to be sure that all is understood by everyone, making sure no one has to do any real thinking? Who does that help? The more I make them think, the more frustration I cause. I know it is not a bad thing though. I know I’m succeeding at my job. Math is supposed to be thought-provoking. I know I might never be appreciated, or I won’t be while these students still are in my class (they are seniors and won’t be in my class again after January). I know I am doing what’s best for them, and that might be why it is so difficult for them and me. They are a lot like my own children, doing what is most difficult for them is doing what is right. But I still have to ask myself, am I doing all I can for my students? How will I know?!

I think a tweet from @misterpatterson sums up my post:

New poster for my classroom. Starting quarter 2 with some focus! pic.twitter.com/sGLIFop8Mw

— Zack Patterson (@misterpatterson) October 27, 2013

Love this and am going to use it in my classroom! Thank you!!

MTBoS Mission #3

This week’s mission was titled – Collaboration Nation. What a great mission! First of all, it exposed me to may sites I have not yet found. It also gave me a chance to look at some sites I saw or have heard of before, but hadn’t had the moment to explore further than the link I clicked on from twitter, or from someone else’s blog. Desmos is something I’d like to look further into because I’ll soon be working on Conic Sections with students. It looks to be a great place to have students create some fabulous artwork with math! There are many sites I am going to try to look at even more, but for this mission, I think I’ll focus on @fawnpnguyen’s VisualPatterns.

Soon, my Honors Precalculus course will be doing a unit on Sequences and Series. Students are typically good at this chapter, as a lot of it is review from Honors Advanced Algebra. This group of students are also good at following the process for writing the rules (equations) and can even distinguish between types of patterns (arithmetic, geometric…). That is the case when numbers are involved. When the numbers are gone, and things get more visual, the patterns tend to be a bit trickier for these kids to battle. I like the idea of giving them a pattern or two to think about at the beginning of class, to get their mind moving. I particularly like the patterns I found at the front of the page when I clicked the link, the pattern that was missing terms in between, but also asked about a 43rd term of the pattern. There are different thought processes that have to go on with something like this, including how the picture should look.

There are also a lot of Vi Hart posts (YouTube) that deal with patterns, and thinking about them differently that I like to show to my class. I am happy to have another resource to share with my students to think about math differently than the “training for Calculus” we typically do throughout their high school experience.

MTBoS – Mission #1

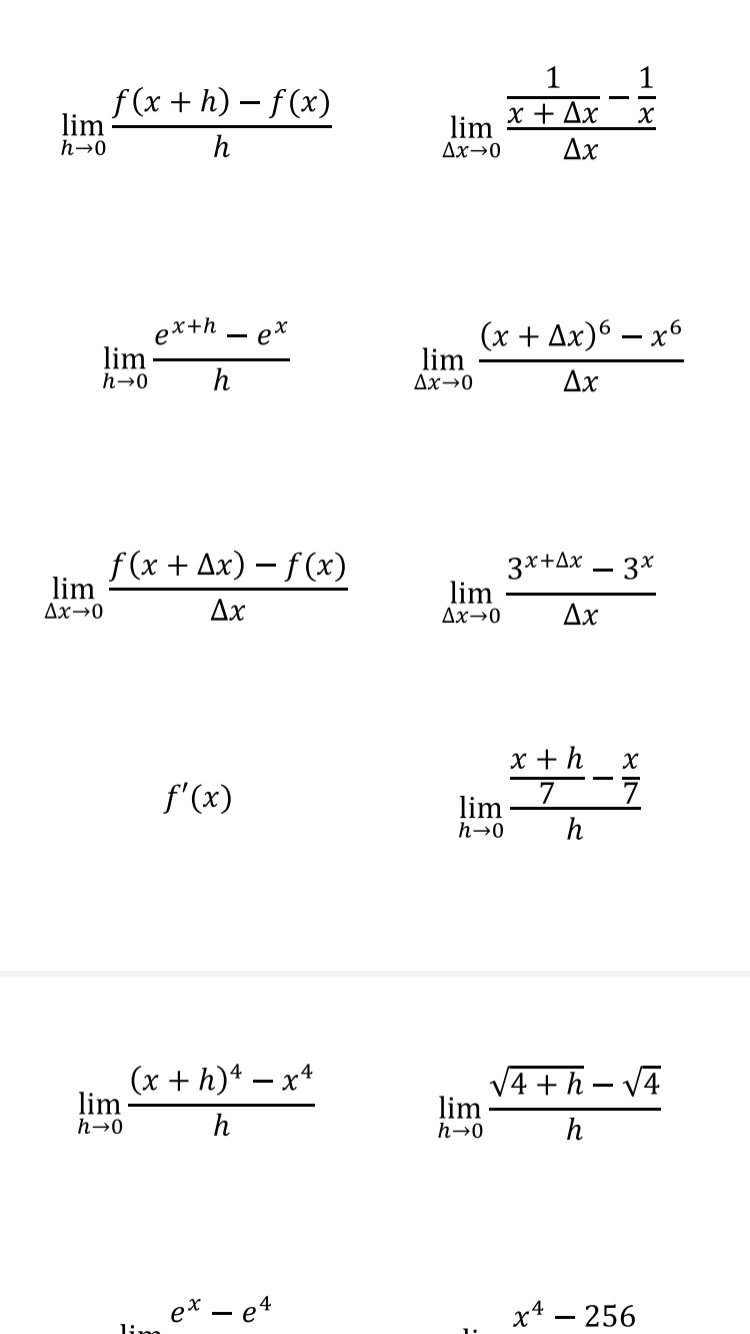
I woke up this morning to my first mission in the Explore the Mathtwitterblogosphere Challenge! Then I read the first mission, and got a bit nervous. I couldn’t think of anything, off the top of my head, that would go along with either topic! Then again, it was 8:30 on a Sunday morning…I had to knock the cob webs out of my head. Then it hit me, Unit Circle Madness! My posting is in response to the topic:

What is one thing that happens in your classroom that makes it distinctly yours? It can be something you do that is unique in your
school… It can be something more amorphous… However you want to interpret the question! Whatever!

My “thing” is not something that happens each day, or each week…and now that I think about it, since I am not teaching honors trig this year for the first time in, well, forever, it might not even go on this year (or will I). But I digress. Unit Circle Madness first came about around the start of a March Madness, the NCAA basketball tournament. I love March Madness, I love trig and the unit circle, why not put them together?!

At the beginning of the week, I introduce students to the idea of the unit circle, we discuss how it’s related to the special triangles, which they already know about, and the trig ratios. We go through and fill out the entire unit circle, angles in degrees and radians, x and y coordinates. I then go through a few tips and tricks for remembering this diagram and all that is on it and tell them that they have to memorize this. There are very few things I think students have to memorize in my classes. The unit circle is one of them because of all the information it contains, its uses for the rest of the course, and the fact that these students will be in AP Calculus and the teachers in those classes expect the students to have knowledge of the unit circle. After going through the entire circle and discussion, I tell the students they need to be able to write it out, as fast as they can, angles, coordinates and all by Friday. I tell them we’ll be going through a bracket challenge, head-to-head competition for glory and prizes. They freak out, of course, wondering how they could be expected to know all of that. I tell them we’ll practice in class each day, but they should also practice on their own, at home, in other classes.

The day of the challenge finally arrives, ands it’s all very exciting for the kids. I post the pairings on the projector, and then I hand out a blank bracket. Much like the office pools some people do for fun (of course) I give the class a few minutes to complete their own brackets. I then collect them and whoever has the most points in the end of this will also earn a prize. Then students arrange the desks into head-to-head pairings (which I set up almost randomly) everyone gets a blank circle, and we’re off! The toughest part for me is getting around to each of the pairings to check. If a mistake is made, I just say no and they try to fix their circle. Whoever wins this round, moves onto the next. Everyone is nervous at first, and feverishly writes, the class is actually quiet for this round. Once all the pairings have a winner, we go back to the bracket and see who won. The students who don’t win now become the bracket checkers, and cheerleaders, and of course, they get a consolation prize (usually a piece of candy). Everyone has a favorite, because there is still the chance of winning the bracket challenge. The point possibilities increase based on each round, many points are still to be had! The next pairings get together, new circles are handed out, and it becomes a little more tense in the room. Some students cheer for their wanna-be champion, but the whole class somehow gets into this “mathletic” event. Usually there are 3 or 4 rounds before the championship round. The final round determines our winner. All eyes are on the final pairing (sometimes there are three because of the number of students in the class). They complete the circle for the 4th or 5th time that day, and a winner is awarded. Points are then tallied, and the bracket winner is also rewarded.

One year, the class somehow talked me into going up against the class winner. I don’t know why I said yes to that. I was so nervous…what would happen if I messed it up, and LOST?! I don’t think I’d live that down with that group. Thank goodness I ended up winning, by a matter of seconds! I had created a monster!

In my school, we are on block scheduling, and the students get new classes every semester. I also had to come up with a hook for the challenge in the fall, and luckily our Detroit Tigers have been in the playoffs for the past couple of years in October, so we use that as our hook…though I still treat it like the March Madness brackets. Even the students who are not into sports, learn a thing or two about current events and how some sports crown their champions.

It is a fun day, and what do you know, all of the students know the unit circle, and remember it through AP Calc! I’ve even gotten a few messages from students who were happy on the first day of college math when they were told to know the unit circle, and what do you know, they already knew it…making the first day a little easier for them.

Here is a link to a bracket I use from print your bracket! a website with all kinds of free bracket configurations : bracket